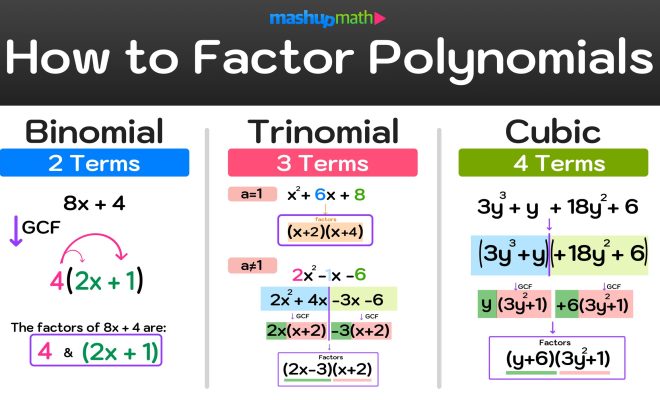
Introduction:

Factoring binomials is an essential skill in algebra and mathematics as a whole. It involves breaking down a binomial expression into the product of its simplest factors. In this article, we will guide you through the process of factoring some common types of binomials, with simple-to-follow steps and examples to ensure you master the technique.

Step 1: Identify the Binomial

A binomial is a mathematical expression that consists of two terms, usually connected by a plus or minus sign. For instance, x^2 + 5x is a binomial.

Step 2: Recognize Basic Binomial Types

There are specific types of binomials that have unique methods for factoring. Some common types include:

1. Difference of Squares: (a^2 – b^2)

2. Perfect Square Trinomal: (a ± b)^2

3. Basic Factoring: ax + bx

Step 3: Factor the Binomial

Now that you have identified the type of binomial, follow these steps to factor it accordingly.

A. Difference of Squares:

When given a binomial like this, (a^2 – b^2), employ the following formula to factor it:

(a + b)(a – b)

Example:

x^2 – 9 can be factored as:

(x + 3)(x – 3)

B. Perfect Square Trinomial:

For a perfect square trinomial, use this method:

(a ± b)^2 ⇒ (a ± b)(a ± b)

Example:

(x + 5)^2 can be factored as:

(x + 5)(x + 5)

C. Basic Factoring:

With basic factoring ax + bx, first determine the greatest common factor (GCF) of both terms:

GCF(a,b)

Then, divide each term by the GCF to get the simplest terms:

ax/GCF(a,b), bx/GCF(a,b)

Finally, factor the binomial by multiplying the GCF by the simplified expression:

GCF(a, b)(x+ (b/GCF(a,b)))

Example:

6x + 9 can be factored as:

3(2x + 3)

Conclusion:

Factoring binomials can be an easy and enjoyable process once you understand the basics. Remember to identify the type of binomial first and apply the appropriate method based on that type. With practice, you’ll soon become adept at factoring binomials with ease.